Descartes' Lost Theorem
 Karl Friedrich Gauss 1777-1855 |
Gaussian curvature. The intrinsic curvature of a surface was defined by Gauss in his General Remarks on Curved Surfaces (1827). For example, at any point in the plane, on a cylinder or on a cone (except the cone point) the Gaussian curvature is 0; at a point on a sphere of radius R, the Gaussian curvature is 1/R2. The Gaussian curvature is an invariant of the local geometry of the surface: that is why it is impossible to make a planar map, with no distorsion, of even a small piece of a spherical surface. |
Gauss' local integral formula. One way of measuring the
Gaussian curvature at a point in a surface is
to take a closed path about the point. The path should be made up of
segments which are as straight as they can be on the surface (``geodesics'');
it can then be described as a geodesic polygon. Gauss tells us
(in slightly different words) that
the sum of the exterior
angles of this polygon is equal to 2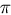 
minus the integral of the
curvature over the enclosed area or, in other terms, to the average
value of the Gaussian curvature inside the polygon times the area
of the polygon. Taking smaller and smaller polygons about the point
will zero in on the exact value of the curvature at the point.
Examples: 1. In the plane, geodesics are ordinary straight lines, and
a geodesic polygon is just a polygon. The sum of the exterior
angles in a polygon is 2 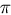: the average value of the Gaussian
curvature inside the polygon is zero.
2. On a sphere, say of radius R, geodesics are great circles.
The equator is a geodesic itself: a geodesic polygon with no exterior
angles. By symmetry the Gaussian curvature must be the same everywhere.
Setting its value times the area of a hemisphere (the ``polygon'' enclosed
by the equator; its area is 2 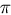R2 ) equal to 2
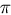gives the curvature as 1/R2.
Polyhedral curvature. Let us apply this same construction on the
surface of a polyhedron. Since the faces are planar, a geodesic polygon
will be made up of straight line segments. Suppose the polygon
encloses a vertex. Then an elementary calculation of angles shows that
the sum of the exterior angles of the polygon is equal to the
sum of the face angles at the vertex. So if the polyhedron had
Gaussian curvature, the integral of that curvature over any polygon
enclosing the vertex would be 2 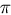minus the sum of the face angles
at that vertex. The only way to interpret this is to say that
(spelling corrected 11/21/14)