Latin Squares in Practice and in Theory II

2. Orthogonal Latin Squares

A latin square of size n is an array of n copies each of n different objects (typically the latin letters A, B, C, ...) so that all the objects in any row, and all the objects in any column, are different. Two size n latin squares, one with objects A, B, C, ..., one with objects a, b, c, are orthogonal if superimposing them leads to a square array containing all n² possibile pairs (A,a), (A,b), ... , (B,a), (B,b), ..., ... .

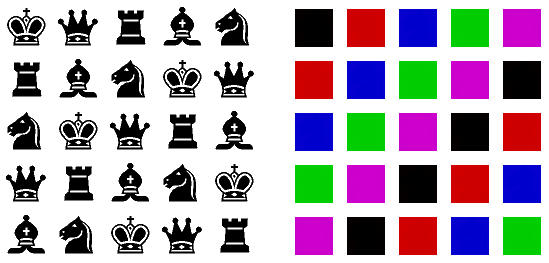
For example, the two 5 x 5 latin squares

are orthogonal: they can be superimposed to give every possible combination of rank and color

If we could find two orthogonal latin squares of size 6, they would combine to give a solution to Euler's problem of the 36 officers. So an equivalent statement to the impossibility of solving that problem is: There are no two orthogonal latin squares of size 6.

1. Leonhard Euler's Puzzle of the 36 Officers
2. Orthogonal latin squares
3. Mutually orthogonal latin squares (MOLS)
4. Orthogonal latin squares and magic squares
5. References in print. For further thought