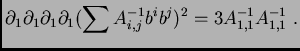Calculating high-order derivatives of a function like
![]() can be very messy. A useful theorem reduces the calculation
to combinatorics.
can be very messy. A useful theorem reduces the calculation
to combinatorics.

Let us calculate a couple of examples.
To begin, it is useful to write
![]() with
with
![]() (the sum running from 1 to
(the sum running from 1 to ![]() ),
using the series expansion
),
using the series expansion
![]() The typical term
will be
The typical term
will be
![]() . This term is a homogeneous polynomial
in the
. This term is a homogeneous polynomial
in the ![]() of degree
of degree ![]() .
.
Differentiating ![]() times a homogeneous polynomial
of degree
times a homogeneous polynomial
of degree ![]() and evaluating at zero will give zero unless
and evaluating at zero will give zero unless ![]() . So the job is to analyze
the result of
. So the job is to analyze
the result of ![]() differentiations on
differentiations on
![]() .
.
The differentiation carried out most frequently in these calculations is
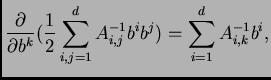
In what follows
![]() will be abbreviated as
will be abbreviated as
![]() .
.
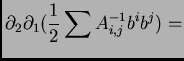
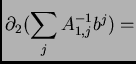
using the symmetry of the matrix ![]() . The same calculation shows
that
. The same calculation shows
that
![]()
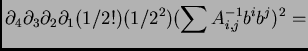
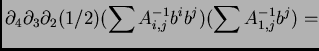
![$\displaystyle \partial_4\partial_3
[(\sum A^{-1}_{2,j}b^j)( \sum A^{-1}_{1,j}b^j) +(1/2)(\sum A^{-1}_{i,j}b^ib^j)A^{-1}_{1,2}] = $](img70.png)
![$\displaystyle \partial_4[A^{-1}_{2,3}( \sum A^{-1}_{1,j}b^j)+(\sum A^{-1}_{2,j}b^j)A^{-1}_{1,3}
+(\sum A^{-1}_{3,j}b^j)A^{-1}_{1,2}] = $](img71.png)
Similarly:
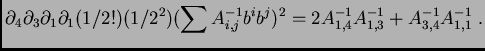

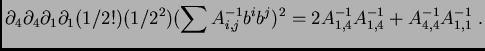
And