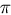Descartes' Lost Theorem

5. Descartes' curvatura

Descartes defines the exterior solid angle at a vertex of a polyhedron as ``that quantity by which the sum of all the plane angles which make up the solid angle is less than four plane right angles,'' i.e. 2 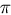minus the sum of the face angles at that vertex, and he states:

Just as in a polygon the sum of the exterior angles is equal to four right angles, so in a polyhedron the sum of the exterior solid angles is equal to 8 solid right angles.

Descartes does not have a completely coherent definition of what a solid right angle should be, and this may be one reason why he never published his Treatise, but computationally he interpreted his statement as meaning:

(Sum of exterior solid angles) = 4 ,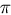

and in this form it is clearly equivalent to his Lost Theorem.

Descartes expands on his definition of exterior angle: ``By exterior angle I mean the curvature and slope of the planes with respect to each other ...'' . In his use of curvatura he foreshadows by two centuries Gauss' definition of the intrinsic curvature of a surface.

• 1. The sum of the plane angles of a polyhedron

• 2. The strange history of Descartes' Treatise

• 3. Euler's Theorems

• 4. The Euler characteristic

• 5. Descartes' curvatura

• 6. Curvature and polyhedral curvature

• 7. The polyhedral Gauss-Bonnet Theorem