
Math and the Musical Offering
4. The transformation
g(t) = f(t-1) + H and Canon 5
In Canon 5 the second voice follows one measure behind, just as
in Canon 2, but it is shifted up in pitch by a perfect fifth.
In Bach's encryption of this canon, the
shift is indicated by the two clefs in the lower staff.
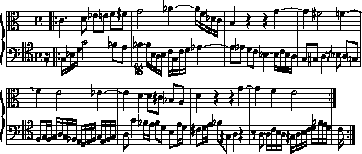
Graphic © 1996,
Timothy A. Smith, used by permission.
The first voice is read
using the bass clef, the second with the same notes but using the alto
clef (centered on middle C). A modified Royal Theme plays in the top staff,
in harmony with the two lower voices.
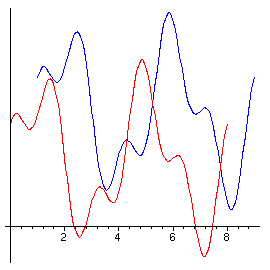 The mathematical analogue of a canon where Voice 2 starts one measure
after Voice 1 and is shifted up a perfect fifth is a pair of functions f(t) and g(t)
with
The mathematical analogue of a canon where Voice 2 starts one measure
after Voice 1 and is shifted up a perfect fifth is a pair of functions f(t) and g(t)
with
g(t) = f(t-1) + H, if the t
unit is a measure,
and where H is the distance along the pitch axis
representing ``up one fifth.''
This canon has another unusual feature, which is not duplicated in the
graphs:
it does not recycle periodically, but each successive run-through is modulated
upward in pitch by a whole step.
© copyright 1999, American Mathematical Society.
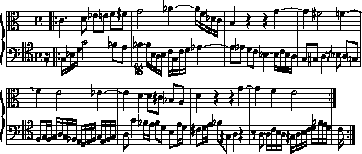

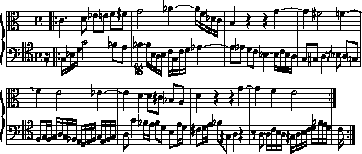
 The mathematical analogue of a canon where Voice 2 starts one measure
after Voice 1 and is shifted up a perfect fifth is a pair of functions f(t) and g(t)
with
The mathematical analogue of a canon where Voice 2 starts one measure
after Voice 1 and is shifted up a perfect fifth is a pair of functions f(t) and g(t)
with