MAT 132 Homework 2 Solutions

Section 6.2

# 2.

use substitution so du = 2x dx;

integral becomes ;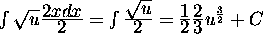

de-substituting gives the answer .

# 7.

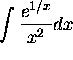
use substitution u=1/x so ;

integral becomes ;

de-substituting gives the answer .

# 12.

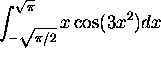
use substitution so du = 6x dx;

indefinite integral becomes ;

transform limits of integration:

becomes , and becomes ;

definite integral becomes .

# 17 (a).

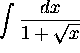
use substitution so , or ;

integral becomes ;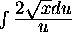

to complete the substitution we need to rewrite the integral completely interms of u;

since , we get ;

the integral now becomes ;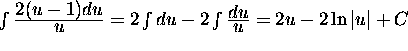

de-substituting gives the answer .(b). Example 7 on p. 409 has .

This is the same as the expression we found here.

#18.

the solution comes from recognizing that this integral looks like ;

get rid of the by writing x as ;

the denominator becomes ;

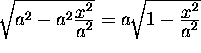now use the substitution u=x/a so du = dx/a;

the integral becomes (notice that we used that a in the denominator!);

de-substituting gives the answer

#22.

(a.) use the substitution , so ;

the integral becomes ;

desubstituting gives the answer .

(b.) use the substitution , so ;

the integral becomes ;

de-substituting gives the answer .

(c.) there is no contradiction because the two C's are not necessarily the same;

since , the first answer can be rewritten as .

More solutions will be posted soon.

• About this document ...