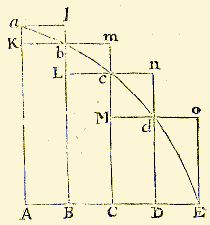
Illustration from Newton's Principia for his
proof that (in modern notation)
for a monotonic function f defined
on an interval [A,E] the difference between
the
upper and lower sums with n equal subdivisions
is equal in absolute value
to
|(f(E)-f(A))(E-A)/n|
(and therefore goes to 0 as n goes to infinity). Here n=4.
Back to MAT 125
|
|