Notes on 2nd order d.e.'s

SUNY at Stony Brook MAT 132 Calculus II

Notes on Second Order Differential Equations
Anthony Phillips

1. The general second order homogeneous linear differential equation with constant coefficients looks like

a₂y'' + a₁y' + a₀y =0,

where y is an unknown function of the variable x. If a₂ =0 this becomes a first order linear equation which we know how to solve. So we will consider the case $a_2 \neq 0$ . Then we can divide through by a₂ and obtain the equivalent equation

y'' + c₁y' + c₀y = 0

with c₁=a₁/a₂ and c₀=a₀/a₂. ``Linear with constant coefficients'' means that each term in the equation is a constant times y or a derivative of y. ``Homogeneous'' excludes equations like y'' + c₁y' + c₀y =f(x) which can be solved, in certain important cases, by an extension of the methods we will study here.

2. The method of solution that we will work on involves looking for an exponential-type solution

$\begin{displaymath}y=e^{\textstyle \lambda x}.\end{displaymath}$

Why? Because it works! We substitute $y=e^{\lambda x}$ in our equation. This gives

$\begin{displaymath}\lambda^2e^{\lambda x}+c_1\lambda e^{\lambda x}+c_0e^{\lambda x}=0.\end{displaymath}$

Since $e^{\lambda x}\neq 0$ we can divide through and get

$\begin{displaymath}\lambda^2 + c_1\lambda + c_0 = 0.\end{displaymath}$

If we solve this quadratic equation for $\lambda$ , then $y=e^{\lambda x}$ will automatically be a solution of our differential equation. So the substitution $y=e^{\lambda x}$ transforms the differential equation into an algebraic equation! This equation is called the characteristic equation (associated to the differential equation).

3. The solution of $a\lambda^2 + b\lambda + c = 0$ is (``quadratic formula'')

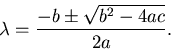
$\begin{displaymath}\lambda = \frac{\textstyle -b\pm\sqrt{b^2-4ac}}{\textstyle 2a}.\end{displaymath}$

In this case a=1, b=c₁, c=c₀, so the solution is

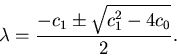
$\begin{displaymath}\lambda = \frac{\textstyle -c_1\pm\sqrt{c_1^2-4c_0}}{\textstyle 2}.\end{displaymath}$

If the discriminant c₁²-4c₀ is positive, then there are two solutions, one for the plus sign and one for the minus.

Example 1. If the differential equation is y''-y=0 (so c₁=0 and c₀=-1) the roots of the characteristic equation $\lambda^2-1=0$ are $\lambda=\frac{\pm\sqrt{4}}{2} = \pm 1$ . Both y=e^x and y=e^-x are solutions. (Check this!)

Example 2. If the differential equation is y''+y'-2y=0 the roots of the characteristic equation $\lambda^2+\lambda-2=0$ are $\lambda=\frac{-1\pm\sqrt{1+8}}{2} = \frac{-1\pm3}{2}$ , so $\lambda_1 = 1$ and $\lambda_2 = -2$ . Both y=e^x and y=e^-2x are solutions. (Check this!)

4. If the discriminant c₁²-4c₀ is negative, then the equation $\lambda^2 + c_1\lambda + c_0 = 0$ has no solutions, unless we enlarge the number field to include $i=\sqrt{-1}$ , i.e. unless we work with complex numbers. If c₁²-4c₀=-k² (we can write any positive number as k²!) then ik is a square root of c₁²-4c₀ since (ik)² = i²k² = (-1)k² = -k². The solutions of the associated algebraic equation are then

$\begin{displaymath}\lambda_1 = \frac{\textstyle -c_1 + ik}{\textstyle 2},~~ \lambda_2 = \frac{\textstyle -c_1 - ik}{\textstyle 2}.\end{displaymath}$

Example 3. If we start with the differential equation y''+y=0 (so c₁=0 and c₀=1) the discriminant is c₁²-4c₀=-4, so 2i is a square root of the discriminant and the solutions of the characteristic equation are $\lambda_1 = i$ and $\lambda_2 = -i$ .

Example 4. If the differential equation is y''+2y'+2y=0 (so c₁=2 and c₀=2 and c₁²-4c₀=4-8 =-4). In this case the solutions of the characteristic equation are $\lambda = (-2\pm2i)/2$ , i.e. $\lambda_1 = -1+i$ and $\lambda_2 = -1-i$ .

5. Going from the solutions of the characteristic equation to the solutions of the differential equation involves interpreting $e^{\lambda x}$ as a function of x when $\lambda$ is a complex number. Suppose $\lambda$ has real part a and imaginary part ib, so that $\lambda = a+ib$ with a and b real numbers. Then

$\begin{displaymath}e^{\lambda x} = e^{(a+ib)x} = e^{ax}e^{ibx}\end{displaymath}$

assuming for the moment that complex numbers can be exponentiated so as to satisfy the law of exponents. The factor e^ax does not cause a problem, but what is e^ibx? Everything will work out if we take

$\begin{displaymath}e^{ibx} = \cos (bx) + i\sin(bx),\end{displaymath}$

and we will see later that this formula is a necessary consequence of the elementary properties of the exponential, sine and cosine functions.

6. Let us try this formula with our examples.

Example 3. For y''+y=0 we found $\lambda_1 = i$ and $\lambda_2 = -i$ , so the solutions are y₁=e^ix and y₂=e^-ix. The formula gives us $y_1=\cos x + i\sin x$ and $y_2=\cos x - i\sin x$ .

Now here is a useful fact about linear differential equations: if y₁and y₂ are solutions of the homogeneous differential equation y''+c₁y'+c₂y=0, then so is the linear combination p y₁ + q y₂ for any numbers p and q. This fact is easy to check (just plug p y₁ + q y₂ into the equation and regroup terms; note that the coefficients a₁ and a₀ do not need to be constant for this to work, that p and q can be complex as well as real constants, and that this works for equations af any order).

Using this fact with the solutions from our example, we notice that $\frac{1}{2}(y_1+y_2) = \cos x$ and $\frac{1}{2i}(y_1-y_2) = \sin x$ are both solutions. When we are given a problem with real coefficients it is customary, and always possible, to exhibit real solutions. Using the fact about linear combinations again, we can say that $y=p\cos x + q\sin x$ is a solution for any p and q. This is the general solution. (It is also correct to call y=p e^ix + q e^-ix the general solution; which one you use depends on the context.)

Example 4. y''+2y'+2y=0. We found $\lambda_1 = -1+i$ and $\lambda_2 = -1-i$ . Using the formula we have

$\begin{displaymath}y_1 = e^{\lambda_1x}=e^{(-1+i) x}=e^{-x}e^{ix}=e^{-x}(\cos x + i\sin x),\end{displaymath}$

$\begin{displaymath}y_2 = e^{\lambda_2x}=e^{(-1-i) x}=e^{-x}e^{-ix}=e^{-x}(\cos x - i\sin x).\end{displaymath}$

Exactly as before we can take $\frac{1}{2}(y_1+y_2)$ and $\frac{1}{2i}(y_1-y_2)$ to get the real solutions $e^{-x}\cos x$ and $e^{-x}\sin x$ . (Check that these functions both satisfy the differential equation!) The general solution will be $y = p e^{-x}\cos x + q e^{-x}\sin x$ .

7. Repeated roots. Suppose the discriminant is zero: c₁²-4c₀ =0. Then the characteristic equation $\lambda^2 + c_1\lambda + c_0 = 0$ has one root. In this case both $e^{\lambda x}$ and $x e^{\lambda x}$ are solutions of the differential equation.

Example 5. Consider the equation y''+4y'+4y=0. Here c₁ = c₀ = 4. The discriminant is $c_1^2-4c_0 = 4^2-4\times 4 = 0$ . The only root is $\lambda = -2$ . Check that both e^-2x and x e^-2x are solutions. The general solution is then y = p e^-2x + q x e^-2x.

8. Initial Conditions. For a first-order differential equation the undetermined constant can be adjusted to make the solution satisfy the initial condition y(0)=y₀; in the same way the p and the q in the general solution of a second order differential equation can be adjusted to satisfy initial conditions. Now there are two: we can specify both the value and the first derivative of the solution for some ``initial'' value of x.

Example 5. Suppose that for the differential equation of Example 2, y''+y'-2y=0, we want a solution with y(0)=1 and y'(0)=-1. The general solution is y=p e^x + q e^-2x, since the two roots of the characteristic equation are 1 and -2. The method is to write down what the initial conditions mean in terms of the general solution, and then to solve for p and q. In this case we have

$\begin{displaymath}1 = y(0)=p e^0 + q e^{-2\times 0} = p + q\end{displaymath}$

$\begin{displaymath}-1 = y'(0)=p e^0 -2 q e^{-2\times 0} = p -2q.\end{displaymath}$

This leads to the set of linear equations p + q = 1, p -2q = -1with solution q = 2/3, p = 1/3. Check that the solution $y = \frac{1}{3}e^x + \frac{2}{3}e^{-2x}$ satisfies the initial conditions.

Example 6. For the differential equation of Example 4, y''+2y'+2y=0, we found the general solution $y = p e^{-x}\cos x + q e^{-x}\sin x$ . To find a solution satisfying the initial conditions y(0)=-2 and y'(0)=1 we proceed as in the last example:

$\begin{displaymath}-2 = y(0) = p e^{-0}\cos 0 + q e^{-0}\sin 0 = p\end{displaymath}$

$\begin{displaymath}1 = y'(0)= -p e^{-0}\cos 0 -p e^{-0}\sin 0 -q e^{-0}\sin 0 + qe^{-0}\cos 0= -p +q.\end{displaymath}$

So p = -2 and q = -1. Check that the solution $y=-2e^{-x}\cos x-e^{-x}\sin x$ satisfies the initial conditions.

Exercises.

Tony Phillips
Math Dept, SUNY at Stony Brook
tony@math.sunysb.edu
November 1 2000